SA2 REVISION QUESTIONS FOR CLASS X

ONE MARK QUESTIONS - CLASS IX

 

IX SA2 PREVIOUS YEAR QUESTION PAPERS

SUMMATIVE ASSESSMENT – II     revision questions

MATHEMATICS             class  IX  

1. Express 5x = 8y, in the form of ax + by + c = 0.

 

2. Find whether line represented by y = 3 passes through origin or not.

 

3. Find the slant height of the cone whose radius is 9 cm and height is 12 cm.
 

4. How much ice-cream can be put into a cone with base radius 3.5 cm and height 12 cm ?

 

5.If two equal chords of a circle intersect within a circle, prove that the segments of a chord are

equal to the corresponding segments of the other chord.

 6. Draw a line segment AB5.6 cm. At point A, construct an angle of 120 , using compass. Now

construct its supplement at the point A.

7. The angles of a quadrilateral are in the ratio 1 : 2 : 2 : 3. Find the angles of the quadrilateral.

What type of quadrilateral is this ?
 

8.The radius and height of a cylinder are in the ratio 5 : 7. If its volume is 4400 cm3, find the

radius of the cylinder.

9.Let x and y be two complementary angles. Form an equation and draw its graph. Find

graphically measure of the other angle , if one of the angle is :
(a) 20 (b) 60

10.Q and R are the centres of two congruent circles intersecting each other at points C and D.

The line joining their centres intersects the circle in points A and B such that A and B do not

 

lie between Q and R. If CD6 cm and AB12 cm, determine the radius of either circle and

the distance between the centres of two circles.

11. Construct PQR, if PQ=7.5 cm, Q 90 and PR RQ 4 cm.

12. Prove that the bisectors of angles of a parallelogram form a rectangle.

13.The students of a school were asked to make and distribute 100 cylindrical penholder of

cardboard with message “Do not use plastic bags” printed on it. Each penholder was to be

of radius 3.5 cm and height 10 cm. The school had to supply the cardboards for this work.

(a) How much cardboard was required ?

(b) What value is depicted in this problem ?

14. Find the length of the cloth 1.1 m wide required to make a conical tent whose height is 12 m

and radius of the base is 5m. Also find the cost of the cloth at the rate of Rs. 70 per sq m.

15. A dome of a building is in the form of a hemisphere. From inside, it was white- washed at

the cost of Rs. 498.96. If the rate of white washing is Rs. 4 per square meter, find the :

(i) Inside surface area of the dome

(ii) Volume of the air inside the dome

16.Draw a line segment PQ of measure 7 cm. Construct its perpendicular bisector and verify it by

actual measurement.

17 .A mansion has 12 cylindrical pillars each having radius 50 cm and height 3.5 m. Find the cost

to paint the curved surface of the pillars at Rs. 20 per square meter.

18.A corn cob, shaped somewhat like a cone has the diameter of its broadest end as 4cm and

length as 20 cm. If each 1 cm2 of the surface of the cob carries an average of three grains, find

how many grains you would find on the entire cob ?
 

 

 

 






 

 

  

 

 

 

 

 

 

  

 

  


 
  

Revision Test no.2

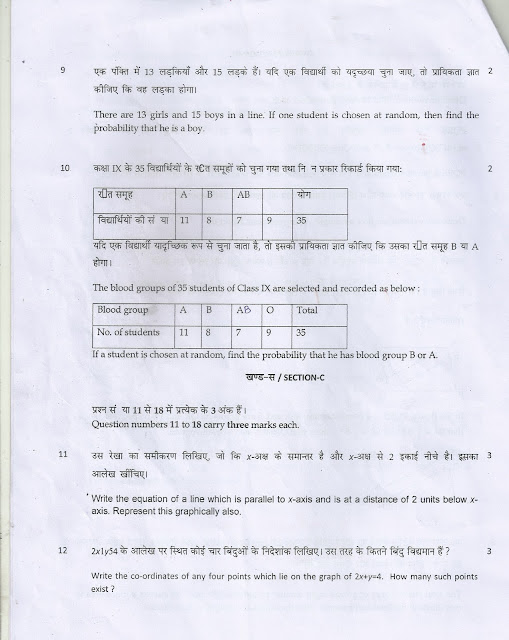
     Revision Test No.2 Chapter - Circles and Probability Time: 1 hour Marks:30
 

 

1.    In the given figure, O is the centre of the circle and ÐBOD=150°. Find ÐBAD and ÐDCP.(2)

2.    To know the opinion of the students about the subject statistics, a survey on 350 students was conducted. The data recorded is as given below :

Opinion      Number of students

Like                  147

Dislike              203

Find the probability that a student chosen at random

(i)            likes statistics. (ii) dislikes statistics.   (2)

3.    Some families with 2 children were selected randomly and the following data were recorded :

Number of girls in a family         0         1          2

Number of families                  184      714      425

If a family is chosen at random, compute the probability that it has

(i)            exactly 1 girl. (ii) exactly 2 boys.    (2)

4.    ·The marks scored by some students in an examination are given in the form of a

frequency distribution table.

Marks :            600-640     640-680   680-720   720-760   760-800   800-840   840-880

No. of Students    16            45            156           284          172           59           18

If a child is selected at random, find the probability that the child :

(i)            scored at least 800 marks (ii) scored at most 680 marks

(iii) has marks between 600  and 880  (3)

5.    In the figure ABCD is a cyclic quadrilateral whose diagonals AC and BD intersect at P. If O is  the centre of the circle and AB = DC, prove that : (i) DPAB @ DPDC  (ii) PA=PD and PC=PB    (iii) AD çç BC  (4)

 

6.    Two dice are thrown simultaneously number of times. Each time the sum of two

numbers appearing on their tops is noted and recorded as given in the following table

Sum of numbers  2      3     4     5     6       7      8      9      10    11     12

Frequency           20   16   28   18    24     22    52   66     48     38     68

If the dice are thrown once more, what is the probability of getting a sum

            (i) which is a multiple of 3 (ii) which is a prime number   (2)

 

7.      In the given figure, O is the centre of the circle. Find the value of x (2)

 

                 

 

8.      The probability of guessing the correct answer to a certain question is  x/3

If the probability of not guessing the correct answer is  5x/3 ,                                     then find the value of x. (2)

9.      The circle passing through the vertices A, B and C of a parallelogram ABCD intersects side CD at a point P as shown in the figure.

 Prove that ∟APD  = ∟ADP. (3)

 

              

10.  In the given figures, BACD is a cyclic quadrilateral in which AC ççBD.

       (i) If ∟BAD=52° and ∟BCA =35°, find ∟ACX.

       (ii) Prove that ∟CBD = ∟ADB. Also deduce that DY = BY.

       (iii)Prove that  ∆XBD is an isosceles triangle.

       (iv) Prove that XA=XC.    (4)

                   

11.  In a bottle there are 7 red buttons, 5 green buttons and 8 purple buttons. What is the probability that randomly drawn button from the bottle is a purple button ? If one extra green button is put in side the bottle, what will be the probability that randomly drawn button is purple ?  (2)

12.  In the given figure, ABCDE is a pentagon. Whose vertices lie on the semicircle with centre O. Find the sum of ∟ACD and ∟DEB ? (2)

 

               

 

 

Revision Test no.1 for class IX

           Revision Test for class IX    marks - 30    Time - 1 hour
Chapter - Constructions,Linear Equation(OTBA) and Areas of Parallelograms and Triangles

1.     Write any one major factor responsible for obesity in children (1) 

2.    How  many  children (below the age of 5)  approximately are overweight in 2010 globally?  (1)

 

3.    In the given figure, ABCD is a parallelogram whose diagonals intersect each other at P. If area of

       parallelogram ABCD is 56 cm², find the area of DAPD (2)

 

                                                

 

4.    If you walk ,you can burn 4 kilo calories/minute, find how  many minutes you have to walk to burn        

       200 kilo calories.(2)

5.      Construct a  DTUV in which   TU+UV+VT = 12.5 cm,  ÐU = 90° and  ÐV = 45° (4)

6.    Find what exercise you have to do and for how many minutes to burn 300 calories and 500 calories (2)

7.    Construct DABC in which ÐA = 60,  AC + BC = 11.5 cm and AB = 4 cm.  (3)

       
       8.    In the figure, PT  çç QR and QT  çç RS. Show that ar (DPQR) =  ar (DQTS). (3)

                    

9.    Draw a line segment AB of measure 6.4 cm. Construct its perpendicular bisector and verify it by

       actual measurement. (3)

10.  PQRS is a parallelogram with diagonals PR and QS intersecting at a point E.

 If ar (∆SEP)  +  ar (∆QER)  =  12 cm², find area of parallelogram PQRS. (3)

        

11.  Construct any obtuse angle. Divide it into four equal parts, using ruler and

compass. (2)

12.  Body mass index is a person’s weight in kilograms divided by the square of height in metres.

       Taking height as 160 cms,  form a linear equation in two variables taking BMI  as x and weight

       as y kg. Draw its graph also. (4)

 

Revision Questions for SA2 class IX 2015-2016

                       

Revision Questions  for class IX

1)      Find the length of the longest iron rod that can be placed in a room 30 m long,  24 m broad and 12 .2 m high.

2)      The lateral surface area of a right circular cylinder of height 12 cm is1848 cm². Find the volume of the cylinder.

3)      A mansion has 12 cylindrical pillars each having radius 50 cm and height 3.5 m. Find the cost to paint the curved surface of the pillars at Rs. 20 per square meter.

4)      Ramesh threw a party on the recovery of his injured friend from the accident. Ramesh served him and  other friends with chilled juice which was in cylindrically shaped cans of radius 4.2 cm   and height 15 cm. Find the total volume of juice they drink and total surface area of 7 juice cans. Which value is depicted by Ramesh ?

5)      A cubicle water tank is filled by tap water at the rate of 1.4 litres per second. Find the length of an edge of the tank in centimeters if the tank is completely filled in 28 minutes.

6)      A closed wooden box has outer dimensions as 10 cm by 8 cm by7 cm. The thickness of wood is 1cm. If 1 cm³ of wood costs Rs 2, find the total cost of the wood required to make the box.

7)      If the total surface area of the sphere is 5544 cm², find the diameter of the sphere.

8)      A hemispherical bowl is made of steel 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.

9)      The patients in a hospital are given soup daily in a cylindrical bowl of diameter 21 cm. On a particular day, the girl decided to cook the soup for patients. If they fill the bowl with soup to a height of 7 cm. (a)Then, how much soup is prepared for 300 patients. (b)    which value is depicted by the girl ?

10)  A corn cob, shaped somewhat like a cone has the diameter of its broadest end as 4cm and length as 20 cm. If each 1 cm²  of the surface of the cob carries an  average of three grains, find how many grains you would find on the entire cob

11)  A heap of wheat is in the form of a cone whose diameter is 10.5 m and height is 3 m. Find its volume. If cost of 1 m³ wheat is Rs.10 then find total cost. Also find slant height of heap.

12)  ABC is an isosceles triangle with each equal  side 5 cm, perimeter 18 cm and height  AD = 7 cm. Then, find the area of the triangle ABC

13)  The area of a triangle is equal to the area of a  rectangle whose length and breadth are 18 cm and 12 cm respectively. If the base of the  triangle is 24 cm, then find its altitude

14)  PQRS is a trapezium with PQ||SR. A line parallel to PR intersects PQ at L and QR at M. Prove that  ar (ΔPSL) = ar (ΔPRM).

15)  In the figure, ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ.     If AQ intersects DC at P, show that ar (BPC) =  ar (DPQ)

16)  Prove that parallelograms on the same base and between the same parallels are equal in area.

17)  ABCD is a trapezium with AB||DC. A line parallel  to AC intersects AB at X and BC at Y.  Prove that ar (ADX) = ar (ACY).

18)  Diagonals of a parallelogram ABCD intersect at point O. Thourgh O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.

19)  Diagonals PR and QS of quadrilateral PQRS intersect at T such that   PT =TR. If           PS = QR, Show that ar (ΔPTS) =  ar (ΔRTQ).

20)  Diagonal AC and BD of a quadrilateral ABCD intersect at O in such a way   that   ar (AOD)= ar (BOC). Prove that ABCD is a trapezium.

21)  PQRS and ABRS are parallelograms and X is any point on side BR. Show that :

i)                    area PQRS = area ABRS

ii)                       area AXS = ½ area PQRS.